
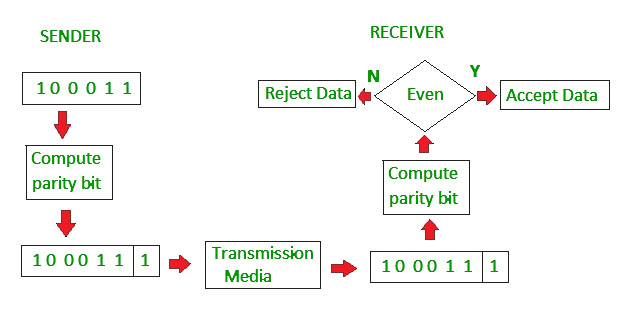
Checksum parity hamming-code data-link-layer error-detection-correction data-communication cyclic-redundancy-check logical-link-control 2d-parity Updated Apr 30, 2018 Java. Which of the following statements is true about a two-dimensional parity check (2D-parity) computed over a payload? 2D-parity can detect any case of a single bit flip in the payload. 2D-parity can detect and correct any case of a single bit flip in the payload. Drawbacks. Both simple parity and 2D parity do not catch all the errors. Simple parity only catches odd numbers of bit errors, and can not catch if 2 bits interchanged. 2D parity is better at catching errors, but requires too many check bits added to a block of data.
- Trending Categories
- Selected Reading

In this article, we will be discussing a program to find the parity of a given number N.
Parity is defined as the number of set bits (number of ‘1’s) in the binary representation of a number.
If the number of ‘1’s in the binary representation are even, the parity is called even parity and if the number of ‘1’s in the binary representation is odd, the parity is called odd parity. Bodi puja gatha sha kavi mp3 downlod.
If the given number is N, we can perform the following operations.
- y = N ^ (N >> 1)
- y = y ^ (y >> 2)
- y = y ^ (y >> 4)
- y = y ^ (y >> 8)
- y = y ^ (y >> 16)

Once all these operations are done, the rightmost bit in y will represent the parity of the number. If the bit is 1, the parity would be odd and if the bit would be 0, the parity will be even.
2d Parity Check Program Cost
Example
Output
2d Parity Check Program In C
- Related Questions & Answers
2d Parity Check Program Cal
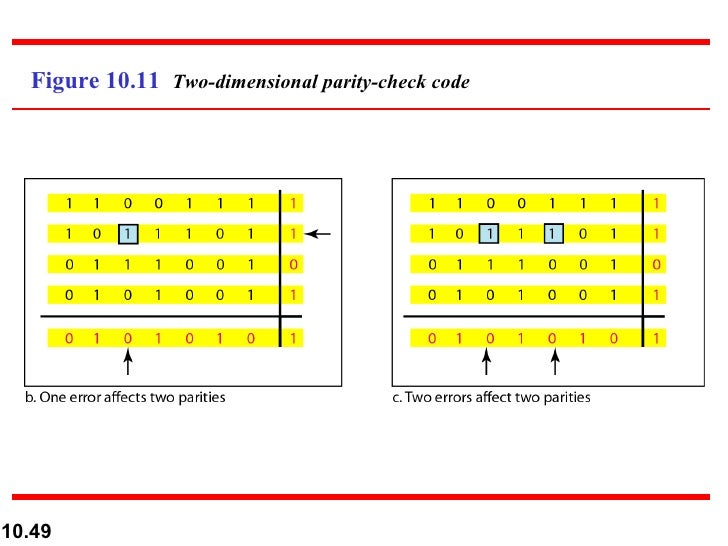
A boolean matrix has the parity property when each row and each column has an even sum, i.e. contains an even number of bits which are set. Here's a 4 x 4 matrix which has the parity property:
1 0 1 0
0 0 0 0
1 1 1 1
0 1 0 1
The sums of the rows are 2, 0, 4 and 2. The sums of the columns are 2, 2, 2 and 2.
Your job is to write a program that reads in a matrix and checks if it has the parity property. If not, your program should check if the parity property can be established by changing only one bit. If this is not possible either, the matrix should be classified as corrupt.
The input file will contain one or more test cases. The first line of each test case contains one integer n (n<100), representing the size of the matrix. On the next n lines, there will be n integers per line. No other integers than 0 and 1 will occur in the matrix. Input will be terminated by a value of 0 for n.
For each matrix in the input file, print one line. If the matrix already has the parity property, print ``OK'. If the parity property can be established by changing one bit, print ``Change bit (i,j)' where i is the row and j the column of the bit to be changed. Otherwise, print ``Corrupt'.